Is the Product of Two Odd Numbers Always Odd

The sum of two odd numbers is always even. The arbitrariness is very important because it means that the final result will be true for any two odd integers we start with.

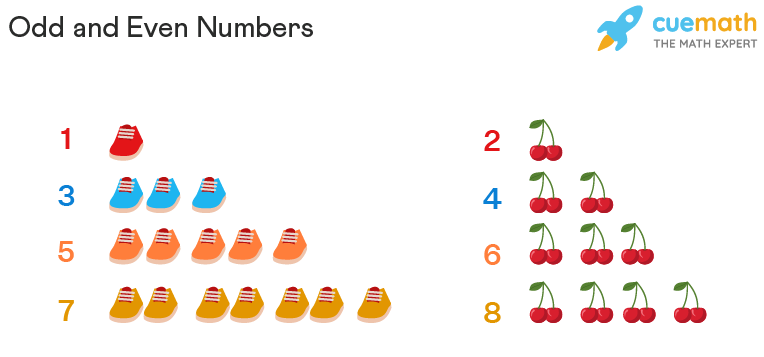
Odd Numbers Definition Properties What Are Odd Numbers

So 2x1 is always odd because adding 1 to an even number is odd.

. If a is odd or even for that matter then a a 2a Because 2 times a number is always even. Show activity on this post. Question a is an odd number.

Show that the product of two odd integers is always odd. The product of two even numbers is even. For example number 3 is an odd number.

A xx b - 1 1 a xx b - 1 1 a xx b - 1 a xx 1 Because b is. The product of an even function and an odd function is an odd function. The product of any two odd numbers is an odd number.

Like given two arbitrary integers a and b. HENCE BY THE ABOVE LOGIC THE PRODUCT IS AN ODD INTEGER. Product of 2 odd integers is 2n1 2m14mn2n2m12 2mnmn12p1 where p is an integer.

The proof lies in recognizing that 2 times an integer is an even integer. That is 2n1 is an odd integer. The product of two odd numbers is an odd number.

Beside above what type of number is the product of two odd numbers. Then this even number N is a multiple of 2. A product of two numbers is either even or odd.

The product of two or more odd numbers is always odd. The number 2 is a prime number. 4 times any number makes a.

What type of number is the product of two odd numbers. Proving That The Product Of Two Different Odd Integers Is Odd. Things to ask yourself.

Is the product of any two prime numbers is always odd. Let m and k be any integers. Answerno this is wrong statement two odd numbers always gives u an even numberexample.

Before we even write the actual proof we need to convince ourselves that the given statement has some truth to it. For the product to be even it has to have a factor of 2. Odd numbers have the digits 1 3 5 7 or 9 in their ones place.

For instance the sum of the four odd numbers 9 13 21 and 17 is 60 while the sum of five odd numbers 7 15 19 23 and 29 is 93. We can test the statement with a few examples. 2N1 IS ODD INTEGER.

So the product of two odd numbers is always odd because 2n 12m 1 22nm n m 1. The product is 4mk 2m 2k 1 hint. 2N1 2M14MN2N2M12 2MNMN12P1 WHERE PIS AN INTEGER.

The sum of two odd numbers is always even. 3 5 15 5 7 35. See an explanation below.

I prepared the table below to gather the results. Same with taking one 2x-1 multiply these. Since 2 divides N it must divide at least one of the factors n or m.

I would then conclude that q r results in an odd number because 2 times an integer with one added to it is by definition an odd number. This means that 2m1 and 2k1 are odd numbers. The sum of two odd numbers is always even.

Yes the product of two odd integers is odd. The same odd number added together will always produce and even number. I used FOIL which can be written as.

If 2 divide n then n is and even number. Let m and n be any integers so that 2m and 2k are two even numbers. We want to show that if we add two odd numbers the sum is always an even number.

It contradicts to the original assumption that n is odd. An odd number is a number that cant be divided by 2. And if it has a factor of two then at least one of the original numbers must have a factor of two and hence is even.

The product of two odd numbers is an odd number. 4 x2 - 1. When you are trying to prove a statement such as The product of two odd numbers is always odd Which of the following is true.

The Sum of Two Odd Numbers is an Even Number. A xx b This can be rewritten as. Number 21 is an odd number.

You can put this solution on YOUR website. The quotient of two even functions is an even function. The sum of an even number of odd numbers is even while the sum of an odd number of odd numbers is odd.

The product means that it is the outcome of a multiplication. 2x1 2x-1 4x2 2x - 2x -1. The product of two or more.

Hence by the above logic the product is an odd integer. The product of two Odd Integers is Odd - YouTube. O al can prove this statement is true by showing a few examples such as 3x395x735 7x1177.

Hali95002 hali95002 10102020 Mathematics High School answered Prove algebraically that the product of two odd numbers is always an odd number. A 538b 13720Step-by-step explanation. If you are trying to predict the product of two evens iseven the product of two odds is odd and the product of an even andan odd is even.

If a and b are odd numbers then we can write their product as. Let assume that the product of two odd numbers m and n is an even number N. Why is the product of two odd functions even.

The product of two or more odd numbers is always odd. Multiplication and division The product of two odd functions is an even function. Let q and r be odd integers then q 2 k 1 and r 2 m 1 where k m Z.

The product of two odd numbers is an odd number. It is always an odd number. IT WILL BE EVEN IF WE MULTIPLY BY 2THAT IS 2N IS EVEN INTEGER.

2 2mk m k 1 which is an odd number. Prove that 3a. Now lets consider the product of our two odd integers 2n12k1 2n cdot 2k 2k 2n 1 4nk2k2n1 22nkkn1 Next you can observe that 2nkkn is some integer lets say m 2nkkn.

If multiplied by another odd number which is number 7 you get the number 21 as a product. The product of two odd counting numbers is always an odd counting number - 14140276. This short video details a small proof showing that the product of two Odd Integers is in fact an Odd Integer.

Patterns With Multiplying Even And Odd Numbers Video Khan Academy

Even And Odd Number Assessment Teaching Math Homeschool Math First Grade Math

Odd Numbers Definition Properties What Are Odd Numbers

Sum Of Two Odd Numbers Is Even Chilimath